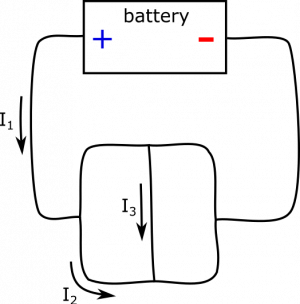
Example: Application of Node Rule
Suppose you have the circuit below. You are given a few values: $I_1=8 \text{ A}$, $I_2=3 \text{ A}$, and $I_3=4 \text{ A}$. Determine all other currents in the circuit, using the Current Node Rule. Draw the direction of the current as well.
Facts
- $I_1=8 \text{ A}$, $I_2=3 \text{ A}$, and $I_3=4 \text{ A}$.
- $I_1$, $I_2$, and $I_3$ are directed as pictured.
- The Node Rule is $I_{in}=I_{out}$, for any point along the current.
Goal
- Find all the currents in the circuit and their directions.
Representations
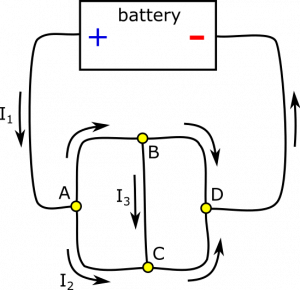
For simplicity of discussion, we label the nodes in an updated representation:
Assumption
We will assume we have a perfect battery to supply a steady current to the circuit and will not die over time.
Solution
Okay, there is a lot going on with all these nodes. Let's make a plan to organize our approach.
Plan
Take the nodes one at a time. Here's the plan in steps:
- Look at all the known currents attached to a node.
- Assign variables to the unknown currents attached to a node.
- Set up an equation using the Node Rule. If not sure about whether a current is going in or coming out of the node, guess.
- Solve for the unknown currents.
- If any of them are negative, then we guessed wrong two steps ago. We can just flip the sign now.
- Repeat the above steps for all the nodes.
Let's start with node $A$. Incoming current is $I_1$, and outgoing current is $I_2$. How do we decide if $I_{A\rightarrow B}$ is incoming or outgoing? We need to bring it back to the Node Rule: $I_{in}=I_{out}$. Since $I_1=8 \text{ A}$ and $I_2=3 \text{ A}$, we need $I_{A\rightarrow B}$ to be outgoing to balance. To satisfy the Node Rule, we set $$I_{A\rightarrow B} = I_{out}-I_2 = I_{in}-I_2 = I_1-I_2 = 5 \text{ A}$$
We do a similar analysis for node $B$. Incoming current is $I_{A\rightarrow B}$, and outgoing current is $I_3$. Since $I_{A\rightarrow B}=5 \text{ A}$ and $I_3=4 \text{ A}$, we need $I_{B\rightarrow D}$ to be outgoing to balance. To satisfy the Node Rule, we set $$I_{B\rightarrow D} = I_{out}-I_3 = I_{in}-I_3 = I_{A\rightarrow B}-I_3 = 1 \text{ A}$$
For node $C$, incoming current is $I_2$ and $I_3$. There is no outgoing current defined yet! $I_{C\rightarrow D}$ must be outgoing to balance. To satisfy the Node Rule, we set $$I_{C\rightarrow D} = I_{out} = I_{in} = I_2+I_3 = 7 \text{ A}$$
Lastly, we look at node $D$. Incoming current is $I_{B\rightarrow D}$ and $I_{C\rightarrow D}$. Since there is no outgoing current defined yet, $I_{D\rightarrow battery}$ must be outgoing to balance. To satisfy the Node Rule, we set $$I_{D\rightarrow battery} = I_{out} = I_{in} = I_{B\rightarrow D}+I_{B\rightarrow D} = 8 \text{ A}$$
Notice that $I_{D\rightarrow battery}=I_1$. This will always be the case for currents going in and out of the battery (approximating a few things that are usually safe to approximate, such as a steady current). In fact, we could have treated the battery as another node in this example. Notice also that if you incorrectly reason about the direction of a current (incoming or outgoing), the calculation will give a negative number for the current. The Node Rule is self-correcting. A final representation with directions is shown below.