Return to Resistors in Series Notes
Example: Resistors in Series
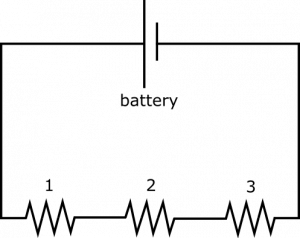
Suppose you have the following circuit. Resistors are labeled 1 through 3 for convenience of reference. You know that the circuit contains a 12-Volt battery, and $R_1=10 \Omega$, $\Delta V_3=6 \text{ V}$, and the power dissipated through Resistor 1 is $P_1 = 0.1 \text{ W}$. What is the resistance of and power dissipated through Resistor 2?
Facts
- $R_1=10 \Omega$
- $\Delta V_3 = 6\text{ V}$
- $\Delta V_{bat} = 12\text{ V}$
- $P_1 = 0.1 \text{ W}$
Lacking
- $R_2$, $P_2$.
Approximations & Assumptions
- The wire has very very small resistance when compared to the other resistors in the circuit: This allows there to be no energy loss across the wires and no potential difference across them either simplifying down the model.
- The circuit is in a steady state: It takes a finite amount of time for a circuit to reach steady state and set up a charge gradient. Making this assumption means the current is not changing with time in any branch of the circuit.
- Approximating the battery as a mechanical battery: This means the battery will supply a steady power source to the circuit to keep it in steady state.
- The resistors in the circuit are made of Ohmic materials: Ohmic materials have a linear relationship between voltage and current, this allows us to use ohms law.
Representations
- We represent Ohm's Law as
\begin{align*} \Delta V = IR &&&&&& (1) \end{align*}
- We represent power dissipated across a potential as
\begin{align*} P = I\Delta V &&&&&& (2) \end{align*}
- We represent the equivalent resistance of multiple resistors arranged in series as
\begin{align*} R_{eq} = R_1+R_2+R_3+\ldots &&&&&& (3) \end{align*}
- We represent the Loop Rule (for potential difference within a closed loop) as
\begin{align*} \Delta V_1+\Delta V_2+\Delta V_3+\ldots = 0 &&&&&& (4) \end{align*}
- We represent the situation with diagram given above.
Solution
Shortly, we will constrain our calculations to just Resistors 1 and 2. We don't have any information on Resistor 2, so our approach will be to find the equivalent resistance of 1 and 2, and then focus on just Resistor 2 using equation (3). The first steps in our approach will be to find the current and potential difference across these two resistors. Note, this is not the only approach that would work! Another way would be to find individual potential difference across each resistor, and then focusing on Resistor 2 from there. (See if you can think of yet another method…)
We can use the Loop Rule – equation (4) – to find the potential difference across these two resistors. The potential difference across the battery has opposite sign as the differences across the resistors, if we consider the circuit as a loop of individual differences. We write: $$\Delta V_{bat} = \Delta V_1 + \Delta V_2 + \Delta V_3$$ Since we know $\Delta V_{bat}$ and $\Delta V_3$, we can plug in and solve: $\Delta V_1 + \Delta V_2 = 6 \text{ V}$.
Plugging equation (1) into the $\Delta V$ of equation (2), we can write the power dissipated through Resistor 1 as $$P_1={I_1}^2R_1$$ Since we know $P_1$ and $R_1$, we can plug in and solve for $I_1=\sqrt{P_1/R_1}=0.1 \text{ A}$. Recall that the Node Rule tells us that the current is the same everywhere in the circuit, since the entire circuit is arranged in a series. so $I=I_1=0.1 \text{ A}$.
We now have enough information to find the equivalent resistance of the two resistors, using Ohm's Law – equation (1). We write: $$R_{\text{1 and 2, equivalent}}=\frac{\Delta V_1 + \Delta V_2}{I}=60 \Omega$$ Now, equation (3) tells us $R_{eq}=R_1+R_2$, so $$R_2=R_{eq}-R_1 = 50\Omega$$ The power dissipated across Resistor 2 can be found using the same rewriting of equation (2) as above: $$P_2=I^2R_2= 0.5 \text{ W}$$
One way in which we can evaluate our solution in this problem is by seeing if the power generated by the battery is equal to the power dissipated through the resistors. You will find that the 1.2 Watts of power generated by the battery is completely dissipated through the resistors. \begin{align*} P_{gen} &= P_{dis} \\ I\Delta V_{bat} &= P_1 + P_2 + P_3 \\ I\Delta V_{bat} &= P_1 + P_2 + I\Delta V_3 \\ 0.1\left(12\right) &= 0.1 + 0.5 + 0.1\left(6\right) \\ 1.2 &= 1.2 \end{align*}