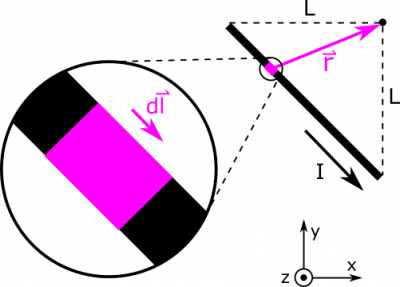
You may have read about how to find the magnetic field from a very long wire of current. Now, what is the magnetic field from a single segment? Suppose we have the configuration shown below. Your observation point is at the origin, and the segment of current $I$ runs in a straight line from $\langle -L, 0, 0 \rangle$ to $\langle 0, -L, 0 \rangle$.

• The current in the segment is $I$.
• The observation point is at the origin.
• The segment stretches from from $\langle -L, 0, 0 \rangle$ to $\langle 0, -L, 0 \rangle$.

• $\vec{B}$

• The current is steady, and the wire segment is uniform.

• We represent the Biot-Savart Law for magnetic field from a current as

$$\vec{B}= \int \frac{\mu_0}{4 \pi}\frac{I \cdot d\vec{l}\times \vec{r}}{r^3}$$

• We represent the situation with diagram given above.

We begin by cracking open the Biot-Savart Law. In order to find magnetic field, we will need to take a cross product $\vec{v}\times \vec{r}$. Notice that $\vec{r}$ indicates a separation vector, directed from source (point particle) to observation (location 1, 2, or 3). Since our source is at the origin of our axes, then the separation vectors are actually just $\vec{r}_{sep} = \vec{r}_{obs} - 0 = \vec{r}_{obs}$, which are just the r-vectors listed in our “Facts”. Below, we have calculated the cross product for each of our three locations: $$\begin{align*} \vec{v}\times \vec{r}_1 &= 0 \\ \vec{v}\times \vec{r}_2 &= 1 \text{ m}^2\text{s}^{-1} \hat{z} \\ \vec{v}\times \vec{r}_3 &= 1 \text{ m}^2\text{s}^{-1} \hat{z} \end{align*}$$ Note that the first cross-product is 0 because location 1 is situated perfectly in line with the straight-line path of the particle. There is no magnetic field at location 1 at all! The other two cross-products are the same because locations 2 and 3 are equidistant from this path. Though they are difference distances from the particle itself, the $\hat{x}$ portion of location 3's separation vector does not contribute at all to the cross-product.

Next, we find the magnitudes of $r^3$, since that is another quantity we need to know in the Biot-Savart Law. $$\begin{align*} {r_2}^3 &= 0.125 \text{ m}^3 \\ {r_3}^3 &= 0.354 \text{ m}^3 \end{align*}$$

We don't including location 1 above since we already know the magnetic field is 0 at that location! Below, we give the magnetic field at all three locations.

$$\begin{align*} \vec{B}_1 &= \frac{\mu_0}{4 \pi}\frac{q\vec{v}\times \vec{r}_1}{{r_1}^3} = 0 \\ \vec{B}_2 &= \frac{\mu_0}{4 \pi}\frac{q\vec{v}\times \vec{r}_2}{{r_2}^3} = 12 \text{ nT } \hat{z} \\ \vec{B}_3 &= \frac{\mu_0}{4 \pi}\frac{q\vec{v}\times \vec{r}_3}{{r_3}^3} = 4.2 \text{ nT } \hat{z} \end{align*}$$